Erfindung von Quadrat von Neun wird William Delbert Gann zugeschrieben, einem berühmten Händler aus der ersten Hälfte des 20. Jahrhunderts, dessen Biografie nicht weniger als sein theoretisches Erbe verbirgt. Forex-Betrüger lieben es, naiven Anfängern das Märchen zu erzählen, wie William Gann an der Börse von Grund auf 50 Millionen Dollar verdiente und die Rentabilität seiner Trades zeitweise 4000 Prozent pro Jahr erreichte! “Mysteriöser Trader”, “unübertroffener Händler”, “Sänger der Spekulation”, “Hüter der heiligen Geheimnisse” – jeder “Börsenevangelist” mit Selbstachtung schreibt solche Beinamen über diesen Patriarchen Wunderbaren Paul.
Grundansicht von Ganns Figur “Quadrat 9”
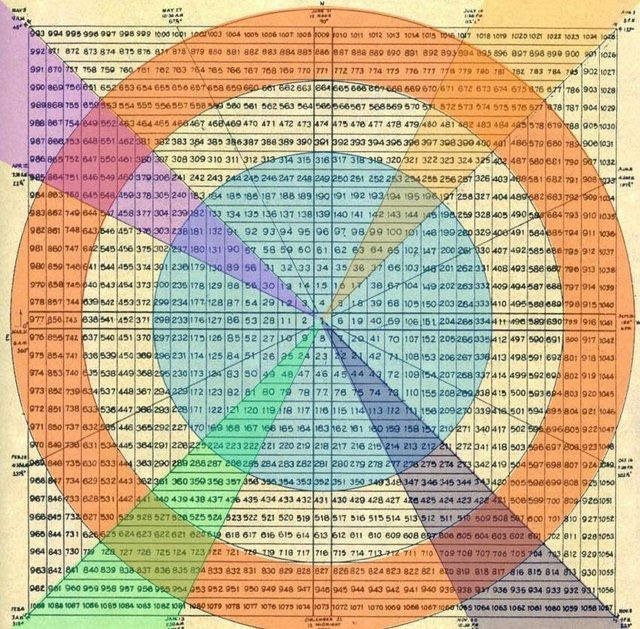
Die Figur „Gann Quadrat von 9“ ist selbst wie eine Pyramide (wenn Sie das Quadrat von oben betrachten). Der Bezugspunkt ist ein kleines zentrales Quadrat, das aus einer Zelle bei Nummer 1 besteht – sozusagen der Spitze der Pyramide. Darüber hinaus gibt es von oben eine Spirale von Zellen mit einer um eine Einheit zunehmenden Anzahl.
Quadrat von 9 erhielt seinen Namen, weil die erste Umdrehung (volle Umdrehung) des Zyklus an der Spitze bei 9 endet.
Was ist laut William Gann die praktische Bedeutung von Square Nine?
Die ursprüngliche Idee folgt natürlich der gleichen heiligen Geometrie, die der Händler aus der Struktur des biblischen Textes ableitet und in seinen berühmten „Ecken“ anwendet: Der sogenannte 1×1-Winkel, der 45 Grad entspricht und als Wasserscheide von Einheiten fungiert: Gut und Böse, Wachstum und Fall, Sonnenaufgang und -untergang usw.
Im Handel entspricht der Haupt-Gann-Winkel (1×1) einem vollwertigen Aufwärtstrend, d. h. einem gesunden Marktwachstum. Die verbleibenden Winkel (1×2, 1×4, 1×8 usw.) dienen als Unterstützungs- und Widerstandsniveaus, ähnlich wie man es bei Fibonacci-Niveaus beobachten kann.
Die Methode Gann Quadrat von neun
Die Zyklen im Gann Quadrat von 9
Wenn Sie mit dem Gann Quadrat von Neun arbeiten, müssen Sie Winkelgrade berechnen. Wir werden diese Grade basierend auf verschiedenen Methoden zur Bestimmung des Zyklus von Quadrat 9 berechnen.
Es gibt sogenannte kleine Zyklen von Quadrat 9. In dem Buch heißt dieses Quadrat Figur 9. Diese ist folgenderweise zu verstehen: der Abstand zwischen den diagonalen Quadraten von ungeraden Zahlen und den diagonalen Quadraten von geraden Zahlen ist 1 gleich. Der Pfad enthält 1/4, ½ und 3/4 Teile (siehe das Bild unten).
Zeichnen wir eine Diagonale durch die Quadrate mit geraden und ungeraden Zahlen (von denen wir oben gesprochen haben), erhalten wir Folgendes: Nehmen wir den Bezugspunkt des Zyklusbeginns auf der Diagonale der ungeraden Zahl (die untere linke Ecke in der Abbildung oben) und das Ende der Referenz auf der Diagonale der geraden Zahlen (in der Abbildung ist dies die obere rechte Ecke), dann können wir den zurückgelegten Weg im Uhrzeigersinn vom Anfang bis zum Endpunkt als ganzen Zyklus darstellen (da wir genau eine Einheit durchlaufen werden – zuerst ¼ des Pfades, dann ½ des Pfades, dann ¾ und schließlich 1 am Ende).
